It’s nearly certain, at this point, that we’ll be able to resume “business as usual” for Fall and act like nothing happened.

Whups, wrong script.

It’s nearly certain, at this point, that even if we resume “business as usual”, it won’t be “business as usual.” Like it or not, online learning and online assessment are here to stay, and COVID-19 only accelerated an existing trend towards distance education. Institutions and faculty who don’t adapt to the new reality are going to be at a competitive disadvantage.

Math is particularly challenging. The existence of “freemium” online problem solvers that show all steps needed to find a solution means that any question that can be answered by following a sequence of steps is meaningless as an assessment instrument. If I ask a student to find

they can use an online problem solver that will also show all the steps; at that point, they just need to cut-and-past the answer. The difficulty is we can’t distinguish a textbook perfect answer generated by a computer algorithm; a textbook perfect answer because they modeled their answer after the textbook perfect examples; and a textbook perfect answer because the student actually listened to us in class. So how can you write problems for the internet age?

We need to constantly ask ourselves: Why did we ask this question?

In this case, the answer might be “To evaluate student understanding of the chain rule.” Leaving aside what we mean by “understanding”, the chain rule is:

and so maybe we want to see if a student understands this concept.

Let’s break this concept down a little further. To correctly apply the chain rule, a student needs to identify an “inside” function and an “outside” function; find the derivatives of both; then use the “inside” function as the argument of the derivative of the “outside” function. The problem is that if we provide a formula for the two functions, an online problem solver can grind through this purely mechanical process.

This means that we can’t provide the formulas.

So how do you ask a math question without providing formulas? Here are some options.

First, if you want a “plug-and-chug” type question (and remember, even good students like these type of questions, because they know what to do with them), you can give partial formulas. For example:

A well-organized student could solve this by following the chain rule, step-by-step. But a problem solver can’t—at least, not yet. (I make no promises for what will happen six months from now)

Online problem solvers are also very bad when it comes to dealing with values from a table, so another possibility is to give a table of values:

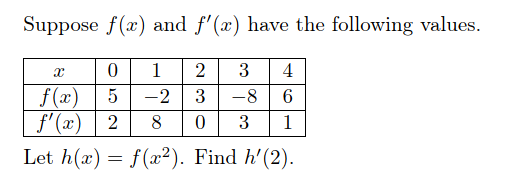
which requires some differentation “by hand”, or

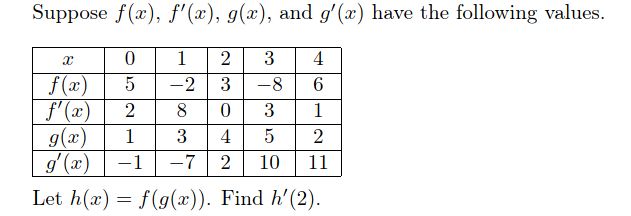
which does not.